CBSE Notes for Class 11 Physics : Measurements and Units

1 Measurement
The process of measurement is basically a comparison process. To measure a physical quantity,

we have to find out how many times a standard amount of that physical quantity is present in the quantity being measured. The number thus obtained is known as the magnitude and the standard

chosen is called the unit of the physical quantity.

2 Unit
The unit of a physical quantity is an arbitrarily chosen standard which is widely accepted by the

society and in terms of which other quantities of similar nature may be measured.

3 Standard

The actual physical embodiment of the unit of a physical quantity is known as a standard of that

physical quantity.
• To express any measurement made we need the numerical value (n) and the unit (μ).

Measurement of physical quantity = Numerical value x Unit
For example: Length of a rod = 8 m
where 8 is numerical value and m (metre) is unit of length.

4 Fundamental Physical Quantity/Units
It is an elementary physical quantity, which does not require any other physical quantity to

express it. It means it cannot be resolved further in terms of any other physical quantity. It is also known as basic physical quantity.
The units of fundamental physical quantities are called fundamental units.
For example, in M. K. S. system, Mass, Length and Time expressed in kilogram, metre and

second respectively are fundamental units.

5 Derived Physical Quantity/Units
All those physical quantities, which can be derived from the combination of two or more fundamental quantities or can be expressed in terms of basic physical quantities, are called derived physical quantities.
The units of all other physical quantities, which car. be obtained from fundamental units, are called derived units. For example, units of velocity, density and force are m/s, kg/m3, kg m/s2 respectively and they are examples of derived units.

6 Systems of Units
Earlier three different units systems were used in different countries. These were CGS, FPS and MKS systems. Now-a-days internationally SI system of units is followed. In SI unit system, seven quantities are taken as the base quantities.
(i) CGS System. Centimetre, Gram and Second are used to express length, mass and time respectively.
(ii) FPS System. Foot, pound and second are used to express length, mass and time respectively.
(iii) MKS System. Length is expressed in metre, mass is expressed in kilogram and time is expressed in second. Metre, kilogram and second are used to express length, mass and time respectively.
(iv) SI Units. Length, mass, time, electric current, thermodynamic temperature, Amount of substance and luminous intensity are expressed in metre, kilogram, second, ampere, kelvin, mole and candela respectively.

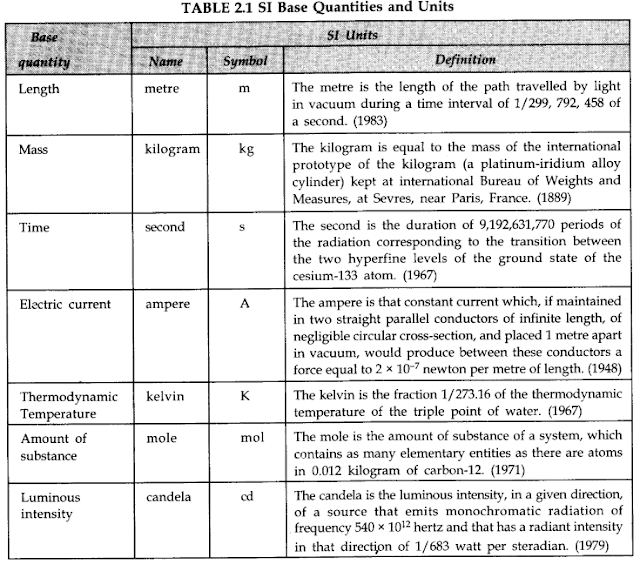
7. Definitions of Fundamental Units

8. Supplementary Units
Besides the above mentioned seven units,there are two supplementary base units. these are (i) radian (rad) for angle, and (ii) steradian (sr) for solid angle.

Advantages of SI Unit System
SI Unit System has following advantages over the other Besides the above mentioned seven units, there are two supplementary base units. These are systems of units:

(i) It is internationally accepted,
(ii) It is a rational unit system,
(iii) It is a coherent unit system,
(iv) It is a metric system,
(v) It is closely related to CGS and MKS systems of units,
(vi) Uses decimal system, hence is more user friendly.
Other Important Units of Length
For measuring large distances e.g., distances of planets and stars etc., some bigger units of length such as ‘astronomical unit’, ‘light year’, parsec’ etc. are used.
• The average separation between the Earth and the sun is called one astronomical unit.
1 AU = 1.496 x 1011 m.
• The distance travelled by light in vacuum in one year is called light year.
1 light year = 9.46 x 1015 m.
• The distance at which an arc of length of one astronomical unit subtends an angle of one second at a point is called parsec.
1 parsec = 3.08 x 1016 m
• Size of a tiny nucleus = 1 fermi = If = 10-15 m
• Size of a tiny atom = 1 angstrom = 1A = 10-10 m

"BOOKS FOR ICSE"

Parallax Method
This method is used to measure the distance of planets and stars from earth.
Parallax. Hold a pen in front of your eyes and look at the pen by closing the right eye and ‘ then the left eye. What do you observe? The position of the pen changes with respect to the background. This relative shift in the position of the pen (object) w.r.t. background is called parallax.
If a distant object e.g., a planet or a star subtends parallax angle 0 on an arc of radius b (known as basis) on Earth, then distance of that distant object from the basis is given by

• To estimate size of atoms we can use electron microscope and tunneling microscopy technique. Rutherford’s a-particle scattering experiment enables us to estimate size of nuclei of different elements.
• Pendulum clocks, mechanical watches (in which vibrations of a balance wheel are used) and quartz watches are commonly used to measure time. Cesium atomic clocks can be used to measure time with an accuracy of 1 part in 1013 (or to a maximum discrepancy of 3 ps in a year).
• The SI unit of mass is kilogram. While dealing with atoms/ molecules and subatomic particles we define a unit known as “unified atomic mass unit” (1 u), where 1 u = 1.66 x 10-27 kg.
Estimation of Molecular Size of Oleic Acid
For this 1 cm3 of oleic acid is dissolved in alcohol to make a solution of 20 cm3. Then 1 cm3 of this solution is taken and diluted to 20 cm3, using alcohol. So, the concentration of the solution is as follows:

After that some lycopodium powder is lightly sprinkled on the surface of water in a large trough and one drop of this solution is put in water. The oleic acid drop spreads into a thin, large and roughly circular film of molecular thickness on water surface. Then, the diameter of the thin film is quickly measured to get its area A. Suppose n drops were put in the water. Initially, the approximate volume of each drop is determined (V cm3).
Volume of n drops of solution = nV cm3
Amount of oleic acid in this solution

The solution of oleic acid spreads very fast on the surface of water and forms a very thin layer of thickness t. If this spreads to form a film of area A cm2, then thickness of the film

If we assume that the film has mono-molecular thickness, this becomes the size or diameter of a molecule of oleic acid. The value of this thickness comes out to be of the order of 10-9 m.
Dimensions
The dimensions of a physical quantity are the powers to which the fundamental units of mass, length and time must be raised to represent the given physical quantity.
Dimensional Formula
The dimensional formula of a physical quantity is an expression telling us how and which of the fundamental quantities enter into the unit of that quantity.
It is customary to express the fundamental quantities by a capital letter, e.g., length (L), mass (AT), time (T), electric current (I), temperature (K) and luminous intensity (C). We write appropriate powers of these capital letters within square brackets to get the dimensional formula of any given physical quantity.
Applications of Dimensions
The concept of dimensions and dimensional formulae are put to the following uses:
(i) Checking the results obtained
(ii) Conversion from one system of units to another
(iii) Deriving relationships between physical quantities
(iv) Scaling and studying of models.
The underlying principle for these uses is the principle of homogeneity of dimensions. According to this principle, the ‘net’ dimensions of the various physical quantities on both sides of a permissible physical relation must be the same; also only dimensionally similar quantities can be added to or subtracted from each other.
Limitations of Dimensional Analysis
The method of dimensions has the following limitations:
(i) by this method the value of dimensionless constant cannot be calculated.
(ii) by this method the equation containing trigonometric, exponential and logarithmic terms cannot be analyzed.
(iii) if a physical quantity in mechanics depends on more than three factors, then relation among them cannot be established because we can have only three equations by equalizing the powers of M, L and T.
(iv) it doesn’t tell whether the quantity is vector or scalar.
Significant Figures
The significant figures are a measure of accuracy of a particular measurement of a physical quantity.
Significant figures in a measurement are those digits in a physical quantity that are known reliably plus the first digit which is uncertain.
The Rules for Determining the Number of Significant Figures
(i) All non-zero digits are significant.
(ii) All zeroes between non-zero digits are significant.
(iii) All zeroes to the right of the last non-zero digit are not significant in numbers without decimal point.
(iv) All zeroes to the right of a decimal point and to the left of a non-zero digit are not significant.
(v) All zeroes to the right of a decimal point and to the right of a non-zero digit are significant.
(vi) In addition and subtraction, we should retain the least decimal place among the values operated, in the result.
(vii) In multiplication and division, we should express the result with the least number of significant figures as associated with the least precise number in operation.
(viii) If scientific notation is not used:
(a) For a number greater than 1, without any decimal, the trailing zeroes are not significant.
(b) For a number with a decimal, the trailing zeros are significant.
Error
The measured value of the physical quantity is usually different from its true value. The result of every measurement by any measuring instrument is an approximate number, which contains some uncertainty. This uncertainty is called error. Every calculated quantity, which is based on measured values, also has an error.
Causes of Errors in Measurement
Following are the causes of errors in measurement:
Least Count Error. The least count error is the error associated with the resolution of the instrument. Least count may not be sufficiently small. The maximum possible error is equal to the least count.
Instrumental Error. This is due to faulty calibration or change in conditions (e.g., thermal expansion of a measuring scale). An instrument may also have a zero error. A correction has to be applied.
Random Error. This is also called chance error. It makes to give different results for same measurements taken repeatedly. These errors are assumed to follow the Gaussian law of normal distribution.
Accidental Error. This error gives too high or too low results. Measurements involving this error are not included in calculations.
Systematic Error. The systematic errors are those errors that tend to be in one direction, either positive or negative. Errors due to air buoyancy in weighing and radiation loss in calorimetry are systematic errors. They can be eliminated by manipulation. Some of the sources of systematic errors are:
(i) intrumental error
(ii) imperfection in experimental technique or procedure
(iii) personal errors
Absolute Error, Relative Error and Percentage Error